 -Groebner Basis Algorithm over PIDs.¶
-Groebner Basis Algorithm over PIDs.¶No attempt was made to optimize this algorithm as the emphasis of this implementation is a clean and easy presentation.
Note
The notion of ‘term’ and ‘monomial’ in [BW93] is swapped from the notion of those words in Sage (or the other way around, however you prefer it). In Sage a term is a monomial multiplied by a coefficient, while in [BW93] a monomial is a term multiplied by a coefficient. Also, what is called LM (the leading monomial) in Sage is called HT (the head term) in [BW93].
EXAMPLE:
sage: from sage.rings.polynomial.toy_d_basis import d_basis
First, consider an example from arithmetic geometry:
sage: A.<x,y> = PolynomialRing(ZZ, 2)
sage: B.<X,Y> = PolynomialRing(Rationals(),2)
sage: f = -y^2 - y + x^3 + 7*x + 1
sage: fx = f.derivative(x)
sage: fy = f.derivative(y)
sage: I = B.ideal([B(f),B(fx),B(fy)])
sage: I.groebner_basis()
[1]
Since the output is 1, we know that there are no generic singularities.
To look at the singularities of the arithmetic surface, we need to do
the corresponding computation over 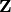 :
:
sage: I = A.ideal([f,fx,fy])
sage: gb = d_basis(I); gb
[x - 2020, y - 11313, 22627]
sage: gb[-1].factor()
11^3 * 17
This Groebner Basis gives a lot of information. First, the only
fibers (over 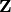 ) that are not smooth are at 11 = 0, and 17 = 0.
Examining the Groebner Basis, we see that we have a simple node in
both the fiber at 11 and at 17. From the factorization, we see that
the node at 17 is regular on the surface (an
) that are not smooth are at 11 = 0, and 17 = 0.
Examining the Groebner Basis, we see that we have a simple node in
both the fiber at 11 and at 17. From the factorization, we see that
the node at 17 is regular on the surface (an 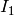 node), but the node
at 11 is not. After blowing up this non-regular point, we find that
it is an
node), but the node
at 11 is not. After blowing up this non-regular point, we find that
it is an 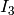 node.
node.
Another example. This one is from the Magma Handbook:
sage: P.<x, y, z> = PolynomialRing(IntegerRing(), 3, order='lex')
sage: I = ideal( x^2 - 1, y^2 - 1, 2*x*y - z)
sage: I = Ideal(d_basis(I))
sage: x.reduce(I)
x
sage: (2*x).reduce(I)
y*z
To compute modulo 4, we can add the generator 4 to our basis.:
sage: I = ideal( x^2 - 1, y^2 - 1, 2*x*y - z, 4)
sage: gb = d_basis(I)
sage: R = P.change_ring(IntegerModRing(4))
sage: gb = [R(f) for f in gb if R(f)]; gb
[x^2 - 1, x*z + 2*y, 2*x - y*z, y^2 - 1, z^2, 2*z]
A third example is also from the Magma Handbook.
This example shows how one can use Groebner bases over the integers to find the primes modulo which a system of equations has a solution, when the system has no solutions over the rationals.
We first form a certain ideal 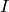 in
in ![\ZZ[x, y, z]](../../../_images/math/03caf5575a323ee1f61c7d917189860582c2d7f0.png) , and note that the
Groebner basis of
, and note that the
Groebner basis of 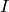 over
over 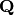 contains 1, so there are no solutions
over
contains 1, so there are no solutions
over 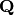 or an algebraic closure of it (this is not surprising as
there are 4 equations in 3 unknowns).:
or an algebraic closure of it (this is not surprising as
there are 4 equations in 3 unknowns).:
sage: P.<x, y, z> = PolynomialRing(IntegerRing(), 3)
sage: I = ideal( x^2 - 3*y, y^3 - x*y, z^3 - x, x^4 - y*z + 1 )
sage: I.change_ring( P.change_ring( RationalField() ) ).groebner_basis()
[1]
However, when we compute the Groebner basis of I (defined over 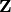 ), we
note that there is a certain integer in the ideal which is not 1.:
), we
note that there is a certain integer in the ideal which is not 1.:
sage: d_basis(I) # random -- waiting on upstream singular fixes at #6051
[x + 170269749119, y + 2149906854, z + ..., 282687803443]
Now for each prime  dividing this integer 282687803443, the Groebner
basis of I modulo
dividing this integer 282687803443, the Groebner
basis of I modulo  will be non-trivial and will thus give a solution
of the original system modulo
will be non-trivial and will thus give a solution
of the original system modulo  .:
.:
sage: factor(282687803443)
101 * 103 * 27173681
sage: I.change_ring( P.change_ring( GF(101) ) ).groebner_basis()
[x + 19, y + 48, z - 33]
sage: I.change_ring( P.change_ring( GF(103) ) ).groebner_basis()
[x + 39, y + 8, z - 18]
sage: I.change_ring( P.change_ring( GF(27173681) ) ).groebner_basis()
[x - 536027, y + 3186055, z + 10380032]
Of course, modulo any other prime the Groebner basis is trivial so there are no other solutions. For example:
sage: I.change_ring( P.change_ring( GF(3) ) ).groebner_basis()
[1]
AUTHOR:
Return the 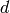 -basis for the Ideal F as defined in [BW93].
-basis for the Ideal F as defined in [BW93].
INPUT:
EXAMPLE:
sage: from sage.rings.polynomial.toy_d_basis import d_basis
sage: A.<x,y> = PolynomialRing(ZZ, 2)
sage: f = -y^2 - y + x^3 + 7*x + 1
sage: fx = f.derivative(x)
sage: fy = f.derivative(y)
sage: I = A.ideal([f,fx,fy])
sage: gb = d_basis(I); gb
[x - 2020, y - 11313, 22627]
Return G-Polynomial of g_1 and g_2.
Let 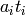 be
be 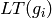 ,
, 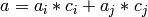 with
with 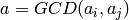 , and
, and  with
with 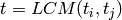 . Then the
G-Polynomial is defined as:
. Then the
G-Polynomial is defined as:  .
.
INPUT:
EXAMPLE:
sage: from sage.rings.polynomial.toy_d_basis import gpol
sage: P.<x, y, z> = PolynomialRing(IntegerRing(), 3, order='lex')
sage: f = x^2 - 1
sage: g = 2*x*y - z
sage: gpol(f,g)
x^2*y - y
The normal selection strategy.
INPUT:
EXAMPLE:
sage: from sage.rings.polynomial.toy_d_basis import select
sage: A.<x,y> = PolynomialRing(ZZ, 2)
sage: f = -y^2 - y + x^3 + 7*x + 1
sage: fx = f.derivative(x)
sage: fy = f.derivative(y)
sage: G = [f, fx, fy]
sage: B = set(filter(lambda (x,y): x!=y, [(f1,f2) for f1 in G for f2 in G]))
sage: select(B)
(3*x^2 + 7, x^3 - y^2 + 7*x - y + 1)
Return S-Polynomial of g_1 and g_2.
Let 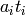 be
be 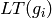 ,
, 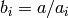 with
with 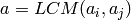 ,
and
,
and  with
with 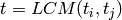 . Then the S-Polynomial
is defined as:
. Then the S-Polynomial
is defined as: 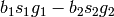 .
.
INPUT:
EXAMPLE:
sage: from sage.rings.polynomial.toy_d_basis import spol
sage: P.<x, y, z> = PolynomialRing(IntegerRing(), 3, order='lex')
sage: f = x^2 - 1
sage: g = 2*x*y - z
sage: spol(f,g)
x*z - 2*y
Update G using the list of critical pairs B and the polynomial h as presented in [BW93], page 230. For this, Buchberger’s first and second criterion are tested.
This function uses the Gebauer-Moeller Installation.
INPUT:
EXAMPLE:
sage: from sage.rings.polynomial.toy_d_basis import update
sage: A.<x,y> = PolynomialRing(ZZ, 2)
sage: G = set([3*x^2 + 7, 2*y + 1, x^3 - y^2 + 7*x - y + 1])
sage: B = set([])
sage: h = x^2*y - x^2 + y - 3
sage: update(G,B,h)
(set([3*x^2 + 7, 2*y + 1, x^2*y - x^2 + y - 3, x^3 - y^2 + 7*x - y + 1]),
set([(x^2*y - x^2 + y - 3, x^3 - y^2 + 7*x - y + 1), (x^2*y - x^2 + y - 3, 3*x^2 + 7), (x^2*y - x^2 + y - 3, 2*y + 1)]))