Generalized Functions¶

Sage implements several generalized functions (also known as distributions) such as Dirac delta, Heaviside step functions. These generalized functions can be manipulated within Sage like any other symbolic functions.

AUTHORS:

Golam Mortuza Hossain (2009-06-26): initial version

EXAMPLES:

Dirac delta function:

sage: dirac_delta(x)
dirac_delta(x)

Heaviside step function:

sage: heaviside(x)
heaviside(x)

Unit step function:

sage: unit_step(x)
unit_step(x)

Signum (sgn) function:

sage: sgn(x)
sgn(x)

Kronecker delta function:

sage: m,n=var('m,n')
sage: kronecker_delta(m,n)
kronecker_delta(m, n)

class sage.functions.generalized.FunctionDiracDelta¶

Bases: sage.symbolic.function.BuiltinFunction

The Dirac delta (generalized) function, 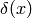$\delta(x)$ (dirac_delta(x)).

INPUT:

x - a real number or a symbolic expression

DEFINITION:

Dirac delta function 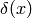$\delta(x)$ , is defined in Sage as:

$\delta(x) = 0$ for real $x \ne 0$ and $\int_{-\infty}^{\infty} \delta(x) dx = 1$

Its alternate definition with respect to an arbitrary test function 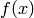$f(x)$ is

$\int_{-\infty}^{\infty} f(x) \delta(x-a) dx = f(a)$

EXAMPLES:

sage: dirac_delta(1)
0
sage: dirac_delta(0)
dirac_delta(0)
sage: dirac_delta(x)
dirac_delta(x)

REFERENCES:

http://en.wikipedia.org/wiki/Dirac_delta_function

class sage.functions.generalized.FunctionHeaviside¶

Bases: sage.symbolic.function.BuiltinFunction

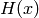The Heaviside step function, $H(x)$ (heaviside(x)).

INPUT:

x - a real number or a symbolic expression

DEFINITION:

The Heaviside step function, 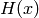$H(x)$ is defined in Sage as:

$H(x) = 0$ for $x < 0$ and $H(x) = 1$ for $x > 0$

EXAMPLES:

sage: heaviside(-1)
0
sage: heaviside(1)
1
sage: heaviside(0)
heaviside(0)
sage: heaviside(x)
heaviside(x)

REFERENCES:

http://en.wikipedia.org/wiki/Heaviside_function

class sage.functions.generalized.FunctionKroneckerDelta¶

Bases: sage.symbolic.function.BuiltinFunction

The Kronecker delta function 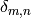$\delta_{m,n}$ (kronecker_delta(m, n)).

INPUT:

m - a number or a symbolic expression
n - a number or a symbolic expression

DEFINITION:

Kronecker delta function 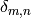$\delta_{m,n}$ is defined as:

$\delta_{m,n} = 0$ for $m \ne n$ and $\delta_{m,n} = 1$ for $m = n$

EXAMPLES:

sage: kronecker_delta(1,2)
0
sage: kronecker_delta(1,1)
1
sage: m,n=var('m,n')
sage: kronecker_delta(m,n)
kronecker_delta(m, n)

REFERENCES:

http://en.wikipedia.org/wiki/Kronecker_delta

class sage.functions.generalized.FunctionSignum¶

Bases: sage.symbolic.function.BuiltinFunction

The signum or sgn function 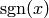$\mathrm{sgn}(x)$ (sgn(x)).

INPUT:

x - a real number or a symbolic expression

DEFINITION:

The sgn function, 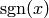$\mathrm{sgn}(x)$ is defined as:

$\mathrm{sgn}(x) = 1$ for $x > 0$ , $\mathrm{sgn}(x) = 0$ for $x = 0$ and $\mathrm{sgn}(x) = -1$ for $x < 0$

EXAMPLES:

sage: sgn(-1)
-1
sage: sgn(1)
1
sage: sgn(0)
0
sage: sgn(x)
sgn(x)

We can also use sign:

sage: sign(1)
1
sage: sign(0)
0
sage: a = AA(-5).nth_root(7)
sage: sign(a)
-1

REFERENCES:

http://en.wikipedia.org/wiki/Sign_function

class sage.functions.generalized.FunctionUnitStep¶

Bases: sage.symbolic.function.BuiltinFunction

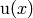The unit step function, $\mathrm{u}(x)$ (unit_step(x)).

INPUT:

x - a real number or a symbolic expression

DEFINITION:

The unit step function, 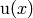$\mathrm{u}(x)$ is defined in Sage as:

$\mathrm{u}(x) = 0$ for $x < 0$ and $\mathrm{u}(x) = 1$ for $x \geq 0$

EXAMPLES:

sage: unit_step(-1)
0
sage: unit_step(1)
1
sage: unit_step(0)
1
sage: unit_step(x)
unit_step(x)

Generalized Functions¶

Previous topic

Next topic

This Page

Navigation

Generalized Functions¶

Previous topic

Next topic

This Page

Quick search

Navigation