AUTHORS:
EXAMPLES:
sage: K.<a,b> = NumberField([x^2 + 1, x^2 + 2])
sage: A = K.absolute_field('z')
sage: I = A.factor(3)[0][0]
sage: from_A, to_A = A.structure()
sage: G = [from_A(z) for z in I.gens()]; G
[3, (-2*b - 1)*a + b - 1]
sage: K.fractional_ideal(G)
Fractional ideal ((b - 1)*a)
sage: K.fractional_ideal(G).absolute_norm().factor()
3^2
Bases: sage.rings.number_field.number_field_ideal.NumberFieldFractionalIdeal
An ideal of a relative number field.
EXAMPLES:
sage: K.<a> = NumberField([x^2 + 1, x^2 + 2]); K
Number Field in a0 with defining polynomial x^2 + 1 over its base field
sage: i = K.ideal(38); i
Fractional ideal (38)
Warning
Ideals in relative number fields are broken:
sage: K.<a0, a1> = NumberField([x^2 + 1, x^2 + 2]); K
Number Field in a0 with defining polynomial x^2 + 1 over its base field
sage: i = K.ideal([a0+1]); i # random
Fractional ideal (-a1*a0)
sage: (g, ) = i.gens_reduced(); g # random
-a1*a0
sage: (g / (a0 + 1)).is_integral()
True
sage: ((a0 + 1) / g).is_integral()
True
If this is an ideal in the extension 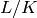 , return the ideal with
the same generators in the absolute field
, return the ideal with
the same generators in the absolute field 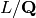 .
.
EXAMPLES:
sage: x = ZZ['x'].0
sage: K.<b> = NumberField(x^2 - 2)
sage: L.<c> = K.extension(x^2 - b)
sage: F = L.absolute_field('a')
An example of an inert ideal:
sage: P = F.factor(13)[0][0]; P
Fractional ideal (13)
sage: J = L.ideal(13)
sage: J.absolute_ideal()
Fractional ideal (13)
Now a non-trivial ideal in 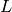 that is principal in the
subfield
that is principal in the
subfield 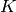 :
:
sage: J = L.ideal(b); J
Fractional ideal (b)
sage: J.absolute_ideal()
Fractional ideal (a^2)
sage: J.relative_norm()
Fractional ideal (2)
sage: J.absolute_norm()
4
sage: J.absolute_ideal().norm()
4
Now an ideal not generated by an element of 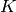 :
:
sage: J = L.ideal(c); J
Fractional ideal (c)
sage: J.absolute_ideal()
Fractional ideal (a)
sage: J.absolute_norm()
2
sage: J.ideal_below()
Fractional ideal (b)
sage: J.ideal_below().norm()
2
Compute the absolute norm of this fractional ideal in a relative number field, returning a positive integer.
EXAMPLES:
sage: L.<a, b, c> = QQ.extension([x^2 - 23, x^2 - 5, x^2 - 7])
sage: I = L.ideal(a + b)
sage: I.absolute_norm()
104976
sage: I.relative_norm().relative_norm().relative_norm()
104976
Return the absolute ramification index of this fractional ideal, assuming it is prime. Otherwise, raise a ValueError.
The absolute ramification index is the power of this prime appearing in the factorization of the rational prime that this prime lies over.
Use relative_ramification_index to obtain the power of this prime occurring in the factorization of the prime ideal of the base field that this prime lies over.
EXAMPLES:
sage: PQ.<X> = QQ[]
sage: F.<a, b> = NumberFieldTower([X^2 - 2, X^2 - 3])
sage: PF.<Y> = F[]
sage: K.<c> = F.extension(Y^2 - (1 + a)*(a + b)*a*b)
sage: I = K.ideal(3, c)
sage: I.absolute_ramification_index()
4
sage: I.smallest_integer()
3
sage: K.ideal(3) == I^4
True
Returns an element  in this ideal such that
in this ideal such that 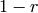 is in other.
is in other.
An error is raised if either ideal is not integral of if they are not coprime.
INPUT:
OUTPUT:
an element  of the ideal self such that
of the ideal self such that 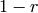 is in the
ideal other.
is in the
ideal other.
EXAMPLES:
sage: K.<a, b> = NumberFieldTower([x^2 - 23, x^2 + 1])
sage: I = Ideal(2, (a - 3*b + 2)/2)
sage: J = K.ideal(a)
sage: z = I.element_1_mod(J)
sage: z in I
True
sage: 1 - z in J
True
Factor the ideal by factoring the corresponding ideal in the absolute number field.
EXAMPLES:
sage: K.<a, b> = QQ.extension([x^2 + 11, x^2 - 5])
sage: K.factor(5)
(Fractional ideal (5, 1/2*a - 1/2*b - 1))^2 * (Fractional ideal (5, 1/2*a - 1/2*b + 1))^2
sage: K.ideal(5).factor()
(Fractional ideal (5, 1/2*a - 1/2*b - 1))^2 * (Fractional ideal (5, 1/2*a - 1/2*b + 1))^2
sage: K.ideal(5).prime_factors()
[Fractional ideal (5, 1/2*a - 1/2*b - 1),
Fractional ideal (5, 1/2*a - 1/2*b + 1)]
sage: PQ.<X> = QQ[]
sage: F.<a, b> = NumberFieldTower([X^2 - 2, X^2 - 3])
sage: PF.<Y> = F[]
sage: K.<c> = F.extension(Y^2 - (1 + a)*(a + b)*a*b)
sage: I = K.ideal(c)
sage: P = K.ideal((b*a - b - 1)*c/2 + a - 1)
sage: Q = K.ideal((b*a - b - 1)*c/2)
sage: list(I.factor()) == [(P, 2), (Q, 1)]
True
sage: I == P^2*Q
True
sage: [p.is_prime() for p in [P, Q]]
[True, True]
Compute the ideal of 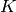 below this ideal of
below this ideal of 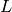 .
.
EXAMPLES:
sage: R.<x> = QQ[]
sage: K.<a> = NumberField(x^2+6)
sage: L.<b> = K.extension(K['x'].gen()^4 + a)
sage: N = L.ideal(b)
sage: M = N.ideal_below(); M == K.ideal([-a])
True
sage: Np = L.ideal( [ L(t) for t in M.gens() ])
sage: Np.ideal_below() == M
True
sage: M.parent()
Monoid of ideals of Number Field in a with defining polynomial x^2 + 6
sage: M.ring()
Number Field in a with defining polynomial x^2 + 6
sage: M.ring() is K
True
This example concerns an inert ideal:
sage: K = NumberField(x^4 + 6*x^2 + 24, 'a')
sage: K.factor(7)
Fractional ideal (7)
sage: K0, K0_into_K, _ = K.subfields(2)[0]
sage: K0
Number Field in a0 with defining polynomial x^2 - 6*x + 24
sage: L = K.relativize(K0_into_K, 'c'); L
Number Field in c0 with defining polynomial x^2 + a0 over its base field
sage: L.base_field() is K0
True
sage: L.ideal(7)
Fractional ideal (7)
sage: L.ideal(7).ideal_below()
Fractional ideal (7)
sage: L.ideal(7).ideal_below().number_field() is K0
True
This example concerns an ideal that splits in the quadratic field but each factor ideal remains inert in the extension:
sage: len(K.factor(19))
2
sage: K0 = L.base_field(); a0 = K0.gen()
sage: len(K0.factor(19))
2
sage: w1 = -a0 + 1; P1 = K0.ideal([w1])
sage: P1.norm().factor(), P1.is_prime()
(19, True)
sage: L_into_K, K_into_L = L.structure()
sage: L.ideal(K_into_L(K0_into_K(w1))).ideal_below() == P1
True
The choice of embedding of quadratic field into quartic field matters:
sage: rho, tau = K0.embeddings(K)
sage: L1 = K.relativize(rho, 'b')
sage: L2 = K.relativize(tau, 'b')
sage: L1_into_K, K_into_L1 = L1.structure()
sage: L2_into_K, K_into_L2 = L2.structure()
sage: a = K.gen()
sage: P = K.ideal([a^2 + 5])
sage: K_into_L1(P).ideal_below() == K0.ideal([-a0 + 1])
True
sage: K_into_L2(P).ideal_below() == K0.ideal([-a0 + 5])
True
sage: K0.ideal([-a0 + 1]) == K0.ideal([-a0 + 5])
False
It works when the base_field is itself a relative number field:
sage: PQ.<X> = QQ[]
sage: F.<a, b> = NumberFieldTower([X^2 - 2, X^2 - 3])
sage: PF.<Y> = F[]
sage: K.<c> = F.extension(Y^2 - (1 + a)*(a + b)*a*b)
sage: I = K.ideal(3, c)
sage: J = I.ideal_below(); J
Fractional ideal (-b)
sage: J.number_field() == F
True
Return a basis for self as a 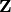 -module.
-module.
EXAMPLE:
sage: K.<a,b> = NumberField([x^2 + 1, x^2 - 3])
sage: I = K.ideal(17*b - 3*a)
sage: x = I.integral_basis(); x # random
[438, -b*a + 309, 219*a - 219*b, 156*a - 154*b]
The exact results are somewhat unpredictable, hence the # random flag, but we can test that they are indeed a basis:
sage: V, _, phi = K.absolute_vector_space()
sage: V.span([phi(u) for u in x], ZZ) == I.free_module()
True
Return a tuple 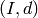 , where
, where 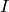 is an integral ideal, and
is an integral ideal, and 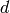 is the
smallest positive integer such that this ideal is equal to
is the
smallest positive integer such that this ideal is equal to 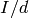 .
.
EXAMPLES:
sage: K.<a, b> = NumberFieldTower([x^2 - 23, x^2 + 1])
sage: I = K.ideal([a + b/3])
sage: J, d = I.integral_split()
sage: J.is_integral()
True
sage: J == d*I
True
Return True if this ideal is integral.
EXAMPLES:
sage: K.<a, b> = QQ.extension([x^2 + 11, x^2 - 5])
sage: I = K.ideal(7).prime_factors()[0]
sage: I.is_integral()
True
sage: (I/2).is_integral()
False
Return True if this ideal of a relative number field is prime.
EXAMPLES:
sage: K.<a, b> = NumberField([x^2 - 17, x^3 - 2])
sage: K.ideal(a + b).is_prime()
True
sage: K.ideal(13).is_prime()
False
Return True if this ideal is principal. If so, set self.__reduced_generators, with length one.
EXAMPLES:
sage: K.<a, b> = NumberField([x^2 - 23, x^2 + 1])
sage: I = K.ideal([7, (-1/2*b - 3/2)*a + 3/2*b + 9/2])
sage: I.is_principal()
True
sage: I # random
Fractional ideal ((1/2*b + 1/2)*a - 3/2*b - 3/2)
Compute the relative norm of this fractional ideal in a relative number field, returning an ideal in the base field.
EXAMPLES:
sage: R.<x> = QQ[]
sage: K.<a> = NumberField(x^2+6)
sage: L.<b> = K.extension(K['x'].gen()^4 + a)
sage: N = L.ideal(b).relative_norm(); N
Fractional ideal (-a)
sage: N.parent()
Monoid of ideals of Number Field in a with defining polynomial x^2 + 6
sage: N.ring()
Number Field in a with defining polynomial x^2 + 6
sage: PQ.<X> = QQ[]
sage: F.<a, b> = NumberField([X^2 - 2, X^2 - 3])
sage: PF.<Y> = F[]
sage: K.<c> = F.extension(Y^2 - (1 + a)*(a + b)*a*b)
sage: K.ideal(1).relative_norm()
Fractional ideal (1)
sage: K.ideal(13).relative_norm().relative_norm()
Fractional ideal (28561)
sage: K.ideal(13).relative_norm().relative_norm().relative_norm()
815730721
sage: K.ideal(13).absolute_norm()
815730721
Return the relative ramification index of this fractional ideal, assuming it is prime. Otherwise, raise a ValueError.
The relative ramification index is the power of this prime appearing in the factorization of the prime ideal of the base field that this prime lies over.
Use absolute_ramification_index to obtain the power of this prime occurring in the factorization of the rational prime that this prime lies over.
EXAMPLES:
sage: PQ.<X> = QQ[]
sage: F.<a, b> = NumberFieldTower([X^2 - 2, X^2 - 3])
sage: PF.<Y> = F[]
sage: K.<c> = F.extension(Y^2 - (1 + a)*(a + b)*a*b)
sage: I = K.ideal(3, c)
sage: I.relative_ramification_index()
2
sage: I.ideal_below()
Fractional ideal (-b)
sage: K.ideal(-b) == I^2
True
Return the residue class degree of this prime.
EXAMPLES:
sage: PQ.<X> = QQ[]
sage: F.<a, b> = NumberFieldTower([X^2 - 2, X^2 - 3])
sage: PF.<Y> = F[]
sage: K.<c> = F.extension(Y^2 - (1 + a)*(a + b)*a*b)
sage: [I.residue_class_degree() for I in K.ideal(c).prime_factors()]
[1, 2]
Returns a iterator through a complete list of residues modulo this integral ideal.
An error is raised if this fractional ideal is not integral.
EXAMPLES:
sage: K.<a, w> = NumberFieldTower([x^2 - 3, x^2 + x + 1])
sage: I = K.ideal(6, -w*a - w + 4)
sage: list(I.residues())[:5]
[(25/3*w - 1/3)*a + 22*w + 1,
(16/3*w - 1/3)*a + 13*w,
(7/3*w - 1/3)*a + 4*w - 1,
(-2/3*w - 1/3)*a - 5*w - 2,
(-11/3*w - 1/3)*a - 14*w - 3]
Return the smallest non-negative integer in 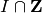 , where
, where 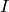 is
this ideal. If
is
this ideal. If 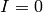 , returns
, returns 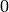 .
.
EXAMPLES:
sage: K.<a, b> = NumberFieldTower([x^2 - 23, x^2 + 1])
sage: I = K.ideal([a + b])
sage: I.smallest_integer()
12
sage: [m for m in range(13) if m in I]
[0, 12]
Return the valuation of this fractional ideal at p.
INPUT:
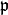 of this relative number field.
of this relative number field.OUTPUT:
(integer) The valuation of this fractional ideal at the prime
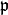 . If
. If 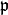 is not prime, raise a
ValueError.
is not prime, raise a
ValueError.
EXAMPLES:
sage: K.<a, b> = NumberField([x^2 - 17, x^3 - 2])
sage: A = K.ideal(a + b)
sage: A.is_prime()
True
sage: (A*K.ideal(3)).valuation(A)
1
sage: K.ideal(25).valuation(5)
...
ValueError: p (= Fractional ideal (5)) must be a prime
Return True if x is a fractional ideal of a relative number field.
EXAMPLES:
sage: from sage.rings.number_field.number_field_ideal_rel import is_NumberFieldFractionalIdeal_rel
sage: from sage.rings.number_field.number_field_ideal import is_NumberFieldFractionalIdeal
sage: is_NumberFieldFractionalIdeal_rel(2/3)
False
sage: is_NumberFieldFractionalIdeal_rel(ideal(5))
False
sage: k.<a> = NumberField(x^2 + 2)
sage: I = k.ideal([a + 1]); I
Fractional ideal (a + 1)
sage: is_NumberFieldFractionalIdeal_rel(I)
False
sage: R.<x> = QQ[]
sage: K.<a> = NumberField(x^2+6)
sage: L.<b> = K.extension(K['x'].gen()^4 + a)
sage: I = L.ideal(b); I
Fractional ideal (b)
sage: is_NumberFieldFractionalIdeal_rel(I)
True
sage: N = I.relative_norm(); N
Fractional ideal (-a)
sage: is_NumberFieldFractionalIdeal_rel(N)
False
sage: is_NumberFieldFractionalIdeal(N)
True