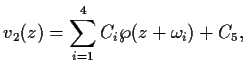In [OOS] we announce a classification of integrable systems invariant
under simple classical Weyl groups.
The precise discussion has already been given by [OS] and [O]
except for the case of type ![]() .
As is shown in [OS], the classification problem for type
.
As is shown in [OS], the classification problem for type ![]() is
reduced to a functional differential equation (1.4).
is
reduced to a functional differential equation (1.4).
In §2 we give a complete list of solutions of this functional equation. Some solutions have already been obtained, after [OP2], by Inozemtsev [IM], [I] (See also [P]). The main result of §2 is Theorem 2.9, which is stated in §1.3 in a different form.
In §3 we examine the reducibility of the system obtained in §2. We note that if the system coincides with the system satisfied by zonal spherical functions of a semisimple Lie group, the reducibility is related to degenerate series representations.
The final draft of this paper was completed when the authors were visiting University of Leiden in the fall of 1994. The authors express their sincere gratitude to Profdrvan Dijk for his hospitality during their stay there.
1.2.
Now we give a quick review of the results in [OS, §6]
concerning with type ![]() .
Let
.
Let ![]() be the Weyl group of type
be the Weyl group of type ![]() , which is identified with
the group of coordinate transformations of
, which is identified with
the group of coordinate transformations of ![]() generated by
generated by
![]() and
and
![]() .
Consider
.
Consider ![]() -invariant differential operators
-invariant differential operators
The operators are proved to be expressed by even functions ![]() and
and ![]() of
one variable as follows ([OS, Proposition 6.3]):
of
one variable as follows ([OS, Proposition 6.3]):
Conversely for any solution ![]() of (1.4) and the pair
of (1.4) and the pair
![]() of
the operators which are given by (1.2) with
of
the operators which are given by (1.2) with
1.3.
We give a complete list of solutions of the functional equation (1.4).
Remind that the Schrödinger operator ![]() is explicitly expressed
as in (1.2) using
is explicitly expressed
as in (1.2) using ![]() and
and ![]() .
.
![]() (Trivial case)
(Trivial case) ![]() constant,
constant, ![]() an arbitrary even function,
an arbitrary even function,
![]()
![]() an arbitrary even function,
an arbitrary even function, ![]() constant.
constant.
Let ![]() and
and ![]() denote the primitive half periods of the Weierstrass elliptic function
denote the primitive half periods of the Weierstrass elliptic function ![]() and put
and put
![]() and
and
![]() .
.
![]() (Elliptic case) For
(Elliptic case) For ![]() ,
,
![]()
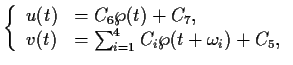 | ||
|
| ||
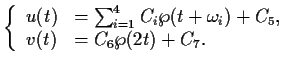 | ||
|
| ||
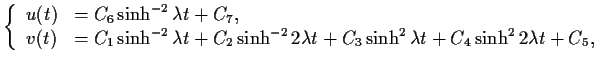 | ||
|
| ||
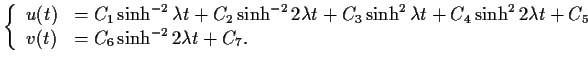 | ||
|
| ||
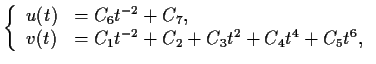 | ||
|
| ||
 | ||
|
| ||
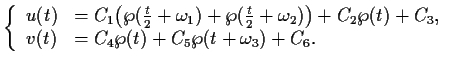 | ||
|
| ||
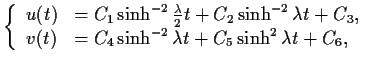 | ||
|
| ||
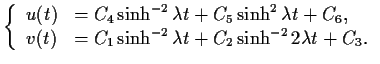 | ||
|
| ||
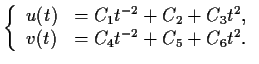 | ||
1.4.
Although we deal with the commuting differential operators of type ![]() with the Weyl group symmetry
in the main body of this paper,
we will give a brief summary of the related works.
with the Weyl group symmetry
in the main body of this paper,
we will give a brief summary of the related works.
The commuting differential operators of type ![]() have been studied very well.
The commuting differential operators of type
have been studied very well.
The commuting differential operators of type ![]() with the Weyl group invariant condition
are classified in [OS].
This work is generalized to
the commuting differential operators of type
with the Weyl group invariant condition
are classified in [OS].
This work is generalized to
the commuting differential operators of type ![]() without Weyl group invariant condition
without Weyl group invariant condition
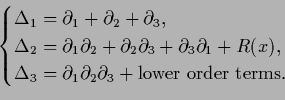
For type ![]() ,
the classification of the commuting differential operators (1.1)
without the Weyl group symmetry has not been done yet.
It is known that the similar functional differential equation
(see (2.3)) is related to such operators.
The following results are obtained in [Oc]:
,
the classification of the commuting differential operators (1.1)
without the Weyl group symmetry has not been done yet.
It is known that the similar functional differential equation
(see (2.3)) is related to such operators.
The following results are obtained in [Oc]:
(i)
We have the expression of the (non Weyl group invariant) operators
![]() and
and ![]() by using four functions
by using four functions
![]() ,
,
![]() ,
,
![]() and
and
![]() with one-variable.
Actually, if we replace
with one-variable.
Actually, if we replace
![]() by
by
![]() ,
,
![]() by
by
![]() , and so on,
the formula (1.2) is also valid for non-invariant operators.
These functions satisfy the functional differential equation like (1.4).
, and so on,
the formula (1.2) is also valid for non-invariant operators.
These functions satisfy the functional differential equation like (1.4).
(ii)
Suppose ![]() be non-trivial (cf. Lemma 2.4 i)).
If
be non-trivial (cf. Lemma 2.4 i)).
If ![]() is holomorphic at some point,
then
is holomorphic at some point,
then ![]() and
and ![]() can be meromorphically continued to
whole plane
can be meromorphically continued to
whole plane
![]() .
The orders of poles of
.
The orders of poles of ![]() are at most two.
are at most two.
(iii)
Suppose, moreover, that ![]() has poles at three points
has poles at three points
![]() such that
such that ![]() and
and ![]() are
linearly independent over
are
linearly independent over
![]() .
Then the function
.
Then the function ![]() can be expressed as
can be expressed as