Introduction

The aim of this paper is to investigate the blowup behavior of solutions to the Brezis-Nirenberg equation with the Robin condition. In our previous paper Kabeya, Yanagida and Yotsutani [10], we proved the range of $\lambda$ for which a unique positive radial solution to

$$\left\{ \begin{array}{ll} &\Delta u+\lambda u+u^{5}=0\quad \... ...partial\nu}}+u=0\quad {\rm on}\ \partial B, \end{array}\right.$$ (1.1)

exists, where $\nu$ is the outward unit normal vector to $\partial B,$ $\lambda<\lambda_0$ ($\lambda_0$ is the first eigenvalue of $-\Delta$ with the homogeneous Robin condition on $B$, $\lambda_0=\pi^2$ if $n=3$ and $\kappa=0$) for each $\kappa\geq 0$.

When $\kappa=0$, in the three dimensional case, a solution to (1.1) exists for $\pi^2/4<\lambda<\pi^2$ while in the higher dimension, a solution does for $0<\lambda<\lambda_0$ (see e.g., Brezis and Nirenberg [4], Brezis and Peletier [5], or [10]). In this sense, the three dimensional case is an exceptional case and interesting phenomena occur in this case. So we concentrate on the three dimensional case.

Since our concern is on radial solutions, we consider the initial value problem of the ordinary differential equation

$$\left\{ \begin{array}{ll} &u_{rr}+\displaystyle{{2\over r}}... ...,\vspace{5pt}\\ &u(0)=\alpha,\ u_{r}(0)=0, \end{array}\right.$$ (1.2)

and seek a suitable number $\alpha>0$ satisfying $u(r)>0$ on $(0,1)$ and

$$\kappa u_r(1)+u(1)=0,$$ (1.3)

where $u_+=\max\{u,0\}$. Note that (1.2) has a solution for any $\alpha>0$ and $\lambda$.

We introduce three numbers. Let $\mu_0=\mu_0(\kappa)\in (0, \pi]$ be defined by

$$\left\{ \begin{array}{lll} &\mu_0=\pi,&{\rm if}\quad \kappa=... ...e{{1\over \kappa},}&{\rm if} \quad\kappa>0. \end{array}\right.$$

Note that $\mu_0^{2}$ is the first radial eigenvalue of $-\Delta$ subject to the boundary condition $\kappa \partial u/\partial \nu+u=0$. For $0\le \kappa \le 1$, define $\mu_1=\mu_{1}(\kappa)\in [0, \pi/2]$ and $\zeta=\zeta(\kappa)\in [0,\infty)$ by

$$\left\{ \begin{array}{lll} &\mu_1=\displaystyle {{\pi\over 2}... ...over \kappa},}&{\rm if} \quad0<\kappa\le 1, \end{array}\right.$$

and

$$\left\{ \begin{array}{lll} &\zeta=\infty,&{\rm if}\quad \kapp... ...ce{5pt}\\ &\zeta=0,&{\rm if}\quad \kappa=1, \end{array}\right.$$

respectively. As we will see in Theorem B, $\lambda=\mu_{1}^2$ is a blowup point. Also note that

$$\left\{ \begin{array}{rl} &\Delta u+\mu_1^2 u=0\ {\rm in }\ B,\vspace{5pt}\\ &\kappa u'(1)+u(1)=0 \end{array}\right.$$

has a positive singular solution.

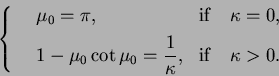
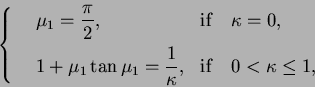
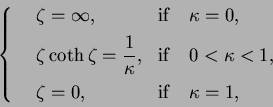
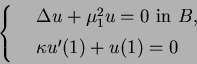
Let us recall our previous results (see also [4] for $\kappa=0$).

Theorem A.(Theorem 1.1 of [10]) Let $n=3$ and $0\leq \kappa\leq 1$. If $\mu_{1}^{2}<\lambda<\mu_{0}^{2}$, then (1.1) has a unique radial solution. If $-\zeta^{2}\leq \lambda\leq \mu_{1}^{2}$, then (1.1) has no radial solution.

By Theorem A, a mapping from $\lambda$ to the initial value $\alpha$ is defined, that is, $\alpha$ is a function of $\lambda\in (\mu_1^2,\mu_0^2]$. Let us denote the unique solution by $u_\lambda$. We can draw the graph of $\alpha=\alpha(\lambda;\kappa)$. Concerning the graph of $\alpha=\alpha(\lambda;\kappa)$, we have the following global behavior.

Theorem B. ((i) of Theorem 1.3 of [10]) Let $0\le \kappa \le 1$. Then the graph of $\alpha(\lambda;\kappa)$ is a continuous curve satisfying $\alpha(\lambda)\to0$ as $\lambda\to\mu_0^{2}-0$ and $\alpha(\lambda;\kappa)\to\infty$ as $\lambda\to\mu_1^{2}+0$.

We can see that $\lambda=\mu_1^{2}$ is the blowup point.

The purpose of this paper is to show the blowup order of $\alpha=\alpha(\lambda;\kappa)$ and an asymptotic behavior of a rescaled solution mainly following the method by Brezis and Peletier [5]. We utilize the Green function as in [5] and Rey [13] used for the Dirichlet problem.

First we consider the case $0\le \kappa <1$.

Theorem 1.1   Let $0\leq \kappa<1$. Then the asymptotic behavior of $\alpha(\lambda;\kappa)$ is

$$\lim_{\lambda\to\mu_1^{2}+0}(\lambda-\mu_1^{2})(\alpha(\lamb... ...mu_1\cos\mu_1\Big\}\over (1-\kappa+\kappa^2\mu_1^2)\sin\mu_1}.$$

The blowup rate of $\alpha(\lambda;\kappa)$ as $\lambda\to\mu_1^{2}+0$ is known by [5] for $\kappa=0$. Not to mention, our results covers that by [5]. In fact, if $\kappa=0$, then $\mu_1=\pi/2$ and the right-hand side is $\sqrt{3}\pi^{3}/2$, which is $\sqrt{3}$ times of that in [5]. Since Brezis and Peletier treated the equation $\Delta \bar u+\lambda \bar u+3\bar u^5=0$, the scaling $u=\sqrt[4]{3}\bar u$ brings this difference. The difference of the coefficient also appears in the limiting behavior of a scaled function (see Theorem 1.3).

In Theorem 1.1, we exclude the case $\kappa=1$. In this case, we see a different blowup order. Note that $\mu_1=0$ when $\kappa=1$. The difference is affected by whether $\mu_1=0$ or not.

Theorem 1.2   When $\kappa=1$, then

$$\lim_{\lambda\to +0}\lambda(\alpha(\lambda;1))^4=3.$$

Similar to [5], the limiting behavior of a scaled function is obtained. Let us denote the Green function of $(-\Delta-\lambda)$ subject to $\kappa \partial u/\partial \nu+u=0$ by $G_{\kappa, \lambda}^{*}(x,y)$ and the ``reduced" Green function $G_{\kappa,\lambda}(x):=G_{\kappa, \lambda}^{*}(x,0)$ for $x\not=0$.

Theorem 1.3   Let $u_\lambda$ be the unique raidial solution to (1.1). Then the asymptotic behavior of $u_\lambda$ is as follows.

(i)

If $0\le \kappa <1$, then

$$\lim_{\lambda \to \mu_1^2+0}{u_\lambda(x) \over \sqrt{\lambd... ...pa) \mu_1\cos\mu_1\Big\}}}G_{\kappa, \mu_1^{2}}(\vert x\vert).$$ (1.4)

for $x\ne 0$.

(ii)

If $\kappa=1$, then

$$\lim_{\lambda\to +0}{u_\lambda(x)\over \sqrt[4]{\lambda}}=4\sqrt[4]{3}\pi G_{1,0}(\vert x\vert)$$ (1.5)

for $x\ne 0$.

Besides $\mu_1=0$ or not, the difference between (i) and (ii) can be explained as the finite part of the reduced Green function $G_{\kappa, \mu_1^2}(\vert x\vert)-1/(4\pi \vert x\vert)\Big\vert _{x=0}$ is nonzero or not. See Theorem 2 of [5] for a similar result on the nearly critical growth.

In [10], the differential form of the Pohozaev identity plays a crucial role. However, to investigate the blowup nature, we need to use the integral form of the identity because it enables us to treat the Dirac $\delta$ function-like behavior.

As related topics, for the Neumann problem ($\kappa=\infty$), such a blowup behavior of solutions to the scalar-field equation with the critical Sobolev exponent is discussed in Budd, Knaap and Peletier [6] and there are many works on the nearly critical growth (see, e.g., Atkinson and Peletier [2], Han [8] or Pan and Wang [12]).

What will happen in the case where $1<\kappa<\infty$? For $\kappa>1$, the unique existence of a solution was also discussed in [10]. Moroever, similar results to Theorems 1.1 and 1.3 are obtained (the blowup rate is as in Theorem 1.1). The blowup point is a continuous function of $\kappa\in[0,\infty]$. These will be discussed in a forthcoming paper [9].

This paper is organized as follows. The Pohozaev identity for an auxiliary inhomogeneous linear problem is discussed in Sections 2 and 3. In Section 4, several properties of the accurately approximate solution, which are useful for proofs of Theorems 1.1, 1.2 and 1.3, are proved. Proofs of Theorem 1.1, 1.2 and 1.3 are given in Section 5.