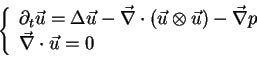
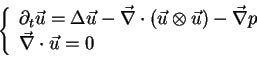
Dealing with the Cauchy problem with initial data
![]() ,
it is possible to rewrite the system
in the following integral expression:
,
it is possible to rewrite the system
in the following integral expression:
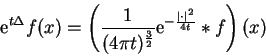
![\begin{displaymath}
\left [
\matrix{
{\rm Id}+R_1R_1 & R_1R_2 & R_1R_3\cr
R_2R_1...
...2 & R_2R_3 \cr
R_3R_1 & R_3R_2 & {\rm Id}+R_3R_3 \cr}
\right ]
\end{displaymath}](img12.png)
In fact the integral and the differential systems are equivalent in this framework, as it is shown in [6] under slight assumptions verified by a large class of spaces.
Given a Banach space ![]() , the problem of the existence and the
uniqueness
of a function
, the problem of the existence and the
uniqueness
of a function
![]() solution of (1) in
solution of (1) in
![]() (a so-called mild solution) is normally approached by a fixed point
argument.
It is now well known ([6], [11], [9]) that for a given
(a so-called mild solution) is normally approached by a fixed point
argument.
It is now well known ([6], [11], [9]) that for a given
![]() the only
solution in
the only
solution in
![]() belongs to every
belongs to every
![]() space with
space with ![]() for all its existence time. We are now interested in investigating the following problem:
if the data
for all its existence time. We are now interested in investigating the following problem:
if the data ![]() have some extra properties, does the solution keep
verifying them at least on a small interval of time?
have some extra properties, does the solution keep
verifying them at least on a small interval of time?
In [6] it was already established that if
![]() ,
the Hardy space, then the solution
,
the Hardy space, then the solution ![]() satisfies
satisfies
![]() for
for ![]() .
We will consider here a particular case of the previous one where the Laplacian
of the data is a ``molecule'' for the Hardy space, and so well-localized
in the space variables; we will prove then that the solution verifies the
same property, namely its diffusion term keeps on being well-localized at the
beginning of the motion.
.
We will consider here a particular case of the previous one where the Laplacian
of the data is a ``molecule'' for the Hardy space, and so well-localized
in the space variables; we will prove then that the solution verifies the
same property, namely its diffusion term keeps on being well-localized at the
beginning of the motion.
In the following the reference space will always be
![]() .
.
3=2 3 by 1 3
More precisely,
let
![]() ,
,
![]() and let us introduce the following functional
space:
and let us introduce the following functional
space:
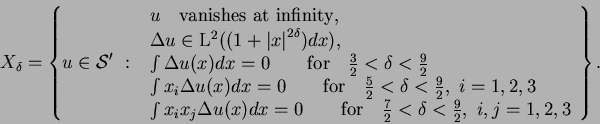
The theorem we are going to prove is the following one.
3=2 3 by 1 3
Theorem .
Let
![]() and
and
![]() Then the mild solution of the Navier-Stokes equations
Then the mild solution of the Navier-Stokes equations
![]() is also in
is also in
![]() for
for ![]() .
.
We would like to stress that the solution we obtain decays pointwise in space like
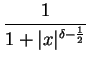 for every
for every ![]() (see also proposition 2).
In the particular case
(see also proposition 2).
In the particular case
![]() , this decay property
may also be recovered by the results of Miyakawa in [12], who establishes a pointwise space-time asymptotic behavior with respect to space-time variables
, this decay property
may also be recovered by the results of Miyakawa in [12], who establishes a pointwise space-time asymptotic behavior with respect to space-time variables ![]() and
and ![]() for a particular class of data.
for a particular class of data.
For other results close to these topics see also [1], [3], [4], [7], [13], [15], [17].